I am struggling with layering and zorder in python. I am making a 3D plot using matplotlib with three relevant elements: A surface_plot of a planet, a surface_plot of rings around that planet, and a contourf image that shows the planet's shadow cast onto the rings.
I want the graphics to display exactly how this scenario would look in real life, with the rings going around the planet and the shadow residing across the rings in the appropriate spot. If the shadow is behind the planet for a given POV, I want the shadow to be blocked by the planet, and vice versa if the shadow is in front of the planet for a given POV.
To be clear, this is ONLY a layering issue. I have the planet, rings, and shadow all plotting correctly. However, the shadow will not ever display in front of the planet. It acts as though the planet is "blocking" the shadow, even though the planet is supposed to be underneath the shadow in terms of layering.
I have tried every single thing I can think of in terms of zorder and rearranging which order the various plot elements are called to be drawn. The rings DO correctly display in front of the planet, but the shadow will not.
My actual code is very long. here are the relevant parts:
Plot setup:
def orthographic_proj(zfront, zback):
a = (zfront+zback)/(zfront-zback)
b = -2*(zfront*zback)/(zfront-zback)
return np.array([[1,0,0,0],
[0,1,0,0],
[0,0,a,b],
[0,0,0,zback]])
def setup_saturn_plot(ax3, elev, azim, drawz, drawxy,view):
#ax3.set_aspect('equal','box')
ax3.view_init(elev=elev, azim=azim)
if(view=="top" or view == "Top" or view == "TOP"):
ax3.dist = 5.5
if(view=="star" or view == "Star" or view == "STAR"):
ax3.dist = 5.0 #4.5 is best value
proj3d.persp_transformation = orthographic_proj
# hide grid and background
ax3.w_xaxis.set_pane_color((1.0, 1.0, 1.0, 1.0))
ax3.w_yaxis.set_pane_color((1.0, 1.0, 1.0, 1.0))
ax3.w_zaxis.set_pane_color((1.0, 1.0, 1.0, 1.0))
ax3.grid(False)
# hide z axis in orthographic top view, xy axes in star view
if (drawz == False):
ax3.w_zaxis.line.set_lw(0.)
ax3.set_zticks([])
if (drawz == True):
ax3.set_zlabel('Z (1000 km)',fontsize=12)
if (drawxy == False):
ax3.w_xaxis.line.set_lw(0.)
ax3.set_xticks([])
ax3.w_yaxis.line.set_lw(0.)
ax3.set_yticks([])
if (drawxy == True):
ax3.set_xlabel('X (1000 km)',fontsize=12)
ax3.set_ylabel('Y (1000 km)',fontsize=12)
Planet:
def draw_saturn(ax3, elev, azim):
# Saturn dimensions
radius = 60268. / 1000.
radius_pole = 54364. / 1000.
# draw Saturn
phi, theta = np.mgrid[0.0:np.pi:100j, 0.0:2.0*np.pi:100j]
x = radius*np.sin(phi)*np.cos(theta)
y = radius*np.sin(phi)*np.sin(theta)
z = radius_pole*np.cos(phi)
line3 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=5, shade=False, lw=0.25)
#line3 = ax3.plot_wireframe(x, y, z, color="w", edgecolor='b', rstride = 5, cstride=5, lw=0.25)
ax3.tick_params(labelsize=10)
rings:
def draw_rings(ax3, elev, azim, draw_mode):
# Saturn dimensions
radius = 60268. / 1000.
# Saturn rings
dringmin = 1.110 * radius
dringmax = 1.236 * radius
cringmin = 1.239 * radius
titanringlet = 1.292 * radius
maxwellgap = 1.452 * radius
cringmax = 1.526 * radius
bringmin = 1.526 * radius
bringmax = 1.950 * radius
aringmin = 2.030 * radius
enckegap = 2.214 * radius
keelergap = 2.265 * radius
aringmax = 2.270 * radius
fringmin = 2.320 * radius
gringmin = 2.754 * radius
gringmax = 2.874 * radius
eringmin = 2.987 * radius
eringmax = 7.964 * radius
if (draw_mode == 'back'):
offset = -azim*np.pi/180. - 0.5*np.pi
if (draw_mode == 'front'):
offset = -azim*np.pi/180. + 0.5*np.pi
rad, theta = np.mgrid[dringmin:dringmax:4j, 0.0-offset:1.0*np.pi-offset:100j]
x = rad * np.cos(theta)
y = rad * np.sin(theta)
z = 0. * rad
line1 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=25, shade=False, lw=0.25,alpha=0.)
rad, theta = np.mgrid[cringmin:cringmax:4j, 0.0-offset:1.0*np.pi-offset:100j]
x = rad * np.cos(theta)
y = rad * np.sin(theta)
z = 0. * rad
line2 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=25, shade=False, lw=0.25,alpha=0.)
rad, theta = np.mgrid[bringmin:bringmax:4j, 0.0-offset:1.0*np.pi-offset:100j]
x = rad * np.cos(theta)
y = rad * np.sin(theta)
z = 0. * rad
line3 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=25, shade=False, lw=0.25,alpha=0.)
rad, theta = np.mgrid[aringmin:aringmax:4j, 0.0-offset:1.0*np.pi-offset:100j]
x = rad * np.cos(theta)
y = rad * np.sin(theta)
z = 0. * rad
line4 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=25, shade=False, lw=0.25,alpha=0.)
rad, theta = np.mgrid[fringmin:1.005*fringmin:2j, 0.0-offset:1.0*np.pi-offset:100j]
x = rad * np.cos(theta)
y = rad * np.sin(theta)
z = 0. * rad
line7 = ax3.plot_surface(x, y, z, color="w", edgecolor='b', rstride = 8, cstride=25, shade=False, lw=0.1,alpha=0.)
Shadow:
def draw_shadowboundary(ax3, sundir):
sqrt = np.sqrt
#azimuthal angle between x direction and direction of sun
alpha = np.arctan2(sundir[1],sundir[0])
#adjustments to keep -pi/2 < alpha < pi/2
alphaadj = 0.*np.pi/180.
if (alpha<0.):
alpha += 2.*np.pi
if ((alpha >= np.pi/2.) & (alpha <= np.pi)):
alpha += np.pi
alphaadj = np.pi
if ((alpha > np.pi) & (alpha <= 3.*np.pi/2.)):
alpha -= np.pi
alphaadj = np.pi
if (alpha>3.*np.pi/2.):
alpha-=2*np.pi
#azimuthal angle between x direction and northern summer -- found using VIMS_2005_14_OMICET and VIMS_2017_053_ALPORI to define eq. of plane of Sun's annual path in chosen coordinate system: -0.193318*x + 0.1963755*y + 0.5471502*z = 0
beta = 44.5505*np.pi/180.
#Saturn's obliquity -- from NASA fact sheet
psi = 26.73*np.pi/180.
#Saturn's oblateness -- from NASA fact sheet
obl = 0.09796
#helpful definitions for optimization
cpsic = np.cos(psi*np.cos(alpha+beta))
spsic = np.sin(psi*np.cos(alpha+beta))
calpha = np.cos(alpha)
salpha = np.sin(alpha)
#Saturn's projected shorter planetary axis as seen by the sun & ring inner edge
req = 60268. / 1000.
b = req*sqrt((1.-obl)*(1.-obl)*cpsic*cpsic + spsic*spsic)
ringstart = 1.239 * req
ringend = 2.270 * req
#shadow boundary of Saturn's rings -- can approximate using a=inf and cancelling terms
a = 9.582*1.496*10.**5
shadowline = lambda x,y : (1/a)*sqrt((req*salpha*(-a+x*calpha*cpsic+y*salpha)*(y*calpha-x*cpsic*salpha)/sqrt((y*calpha-x*cpsic*salpha)**2 + (x*spsic)**2) + calpha*(a*cpsic*(x*calpha*cpsic+y*salpha) + b*x*(a-x*calpha*cpsic-y*salpha)*spsic*spsic/sqrt((y*calpha-x*cpsic*salpha)**2 + (x*spsic)**2)))**2 + (req*calpha*(a-x*calpha*cpsic-y*salpha)*(y*calpha-x*cpsic*salpha)/sqrt((y*calpha-x*cpsic*salpha)**2 + (x*spsic)**2) + salpha*(a*cpsic*(x*calpha*cpsic+y*salpha)+b*x*(a-x*calpha*cpsic-y*salpha)*spsic*spsic/sqrt((y*calpha-x*cpsic*salpha)**2 + (x*spsic)**2)))**2)
#azimuthal radius & antisolar angle for inequalities
radius = lambda x,y : np.sqrt(x**2+y**2)
anti = lambda x,y : abs(np.arctan2(y,x)-(alpha-alphaadj))
#properties of shadow
samples=1200
d = np.linspace(-3*req,3*req,samples)
x,y = np.meshgrid(d,d)
#z = ((radius(x,y)<=shadowline(x,y)) & (ringstart<=radius(x,y)) & (np.pi/2<=anti(x,y)) & (anti(x,y)<=3.*np.pi/2)).astype(int)
z = ((radius(x,y)<=shadowline(x,y)) & (ringstart<=radius(x,y)) & (radius(x,y)<=ringend) & (np.pi/2<=anti(x,y)) & (anti(x,y)<=3.*np.pi/2)).astype(int)
cmap = matplotlib.colors.ListedColormap(["k","k"])
#add shadow to plot
ax3.contourf(x,y,z, [0.5,1.50001], cmap=cmap,alpha=0.5)
Combine graphics:
import matplotlib
import numpy
from math import *
import matplotlib.pyplot as plt
import numpy as np
from mpl_toolkits.mplot3d import Axes3D # <--- This is important for 3d plotting
from mpl_toolkits.mplot3d import proj3d
def plot_results(phi, theta, sundir=[0.5, 0.5]):
#plot_names.append("occultation_track_" + starname)
fig2 = plt.figure(figsize=(9,9))
ax3 = fig2.add_subplot(111, projection='3d')
setup_saturn_plot(ax3, phi, theta, False, False, "star")
draw_saturn(ax3, phi, theta)
draw_rings(ax3, phi, theta, 'back')
draw_rings(ax3, phi, theta, 'front')
draw_shadowboundary(ax3,sundir)
ax3.set_xlim([-200, 200])
ax3.set_ylim([-200, 200])
ax3.set_zlim([-200, 200])
plot_results(phi=40, theta=50, sundir = (30,60))
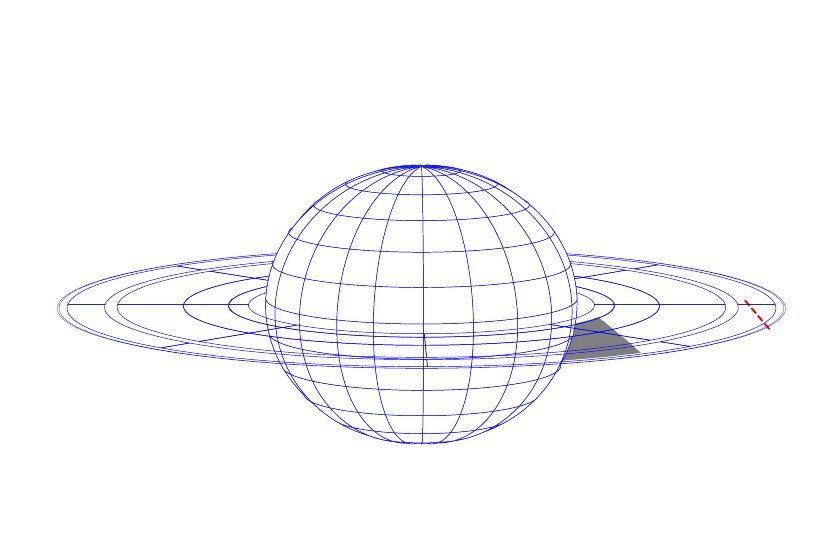
The code produces an image like this:
The grey shadow is supposed to be residing on the rings in front of the planet. However, it won't display in front of the planet, so only the little sliver of shadow to the right of the planet is actually appearing. The shadow displays correctly in all scenarios except when it needs to go in front the planet.
Any fixes for this?