In general, the angle of a vector (x, y) can be calculated by math.atan2(y, x). The vector can be defined by 2 points (x1, y1) and (x2, y2) on a line. Therefore the angle of the line is math.atan2(y2-y1, x2-x1).
Be aware that the y-axis needs to be reversed (-y respectively y1-y2) because the y-axis is generally pointing up but in the PyGame coordinate system the y-axis is pointing down. The unit of the angle in the Python math module is Radian, but the unit of the angle in PyGame functions like pygame.transform.rotate() is Degree. Hence the angle has to be converted from Radians to Degrees by math.degrees:
import math
def angle_of_vector(x, y):
return math.degrees(math.atan2(-y, x))
def angle_of_line(x1, y1, x2, y2):
return math.degrees(math.atan2(-(y2-y1), x2-x1))
This can be simplified by using the angle_to method of the pygame.math.Vector2 object. This method computes the angle between 2 vectors in the PyGame coordinate system in degrees. Therefore it is not necessary to reverse the y-axis and convert from radians to degrees. Just calculate the angle between the vector and (1, 0):
def angle_of_vector(x, y):
return pygame.math.Vector2(x, y).angle_to((1, 0))
def angle_of_line(x1, y1, x2, y2):
return angle_of_vector(x2-x1, y2-y1)
Minimale example:
![]()
import pygame
import math
def angle_of_vector(x, y):
#return math.degrees(math.atan2(-y, x)) # 1: with math.atan
return pygame.math.Vector2(x, y).angle_to((1, 0)) # 2: with pygame.math.Vector2.angle_to
def angle_of_line(x1, y1, x2, y2):
#return math.degrees(math.atan2(-y1-y2, x2-x1)) # 1: math.atan
return angle_of_vector(x2-x1, y2-y1) # 2: pygame.math.Vector2.angle_to
pygame.init()
window = pygame.display.set_mode((400, 400))
clock = pygame.time.Clock()
font = pygame.font.SysFont(None, 50)
angle = 0
radius = 150
vec = (radius, 0)
run = True
while run:
clock.tick(60)
for event in pygame.event.get():
if event.type == pygame.QUIT:
run = False
cpt = window.get_rect().center
pt = cpt[0] + vec[0], cpt[1] + vec[1]
angle = angle_of_vector(*vec)
window.fill((255, 255, 255))
pygame.draw.circle(window, (0, 0, 0), cpt, radius, 1)
pygame.draw.line(window, (0, 255, 0), cpt, (cpt[0] + radius, cpt[1]), 3)
pygame.draw.line(window, (255, 0, 0), cpt, pt, 3)
text_surf = font.render(str(round(angle/5)*5) + "°", True, (255, 0, 0))
text_surf.set_alpha(127)
window.blit(text_surf, text_surf.get_rect(bottomleft = (cpt[0]+20, cpt[1]-20)))
pygame.display.flip()
angle = (angle + 1) % 360
vec = radius * math.cos(angle*math.pi/180), radius * -math.sin(angle*math.pi/180)
pygame.quit()
exit()
angle_to can be used to calculate the angle between 2 vectors or lines:
def angle_between_vectors(x1, y1, x2, y2):
return pygame.math.Vector2(x1, y1).angle_to((x2, y2))
Minimal example:
![]()
import pygame
import math
def angle_between_vectors(x1, y1, x2, y2):
return pygame.math.Vector2(x1, y1).angle_to((x2, y2))
def angle_of_vector(x, y):
return pygame.math.Vector2(x, y).angle_to((1, 0))
pygame.init()
window = pygame.display.set_mode((400, 400))
clock = pygame.time.Clock()
font = pygame.font.SysFont(None, 50)
angle = 0
radius = 150
vec1 = (radius, 0)
vec2 = (radius, 0)
run = True
while run:
clock.tick(60)
for event in pygame.event.get():
if event.type == pygame.QUIT:
run = False
cpt = window.get_rect().center
pt1 = cpt[0] + vec1[0], cpt[1] + vec1[1]
pt2 = cpt[0] + vec2[0], cpt[1] + vec2[1]
angle = angle_between_vectors(*vec2, *vec1)
window.fill((255, 255, 255))
pygame.draw.circle(window, (0, 0, 0), cpt, radius, 1)
pygame.draw.line(window, (0, 255, 0), cpt, pt1, 3)
pygame.draw.line(window, (255, 0, 0), cpt, pt2, 3)
text_surf = font.render(str(round(angle/5)*5) + "°", True, (255, 0, 0))
text_surf.set_alpha(127)
window.blit(text_surf, text_surf.get_rect(bottomleft = (cpt[0]+20, cpt[1]-20)))
pygame.display.flip()
angle1 = (angle_of_vector(*vec1) + 1/3) % 360
vec1 = radius * math.cos(angle1*math.pi/180), radius * -math.sin(angle1*math.pi/180)
angle2 = (angle_of_vector(*vec2) + 1) % 360
vec2 = radius * math.cos(angle2*math.pi/180), radius * -math.sin(angle2*math.pi/180)
pygame.quit()
exit()


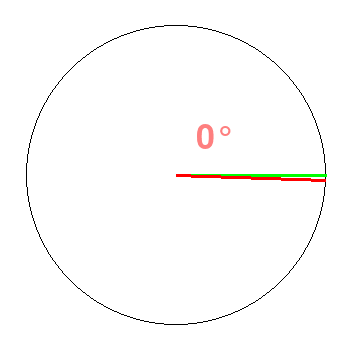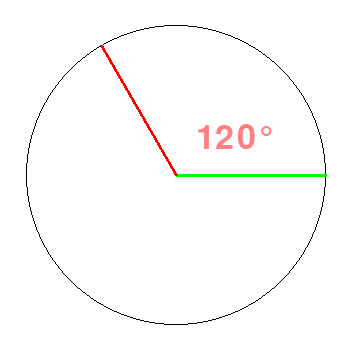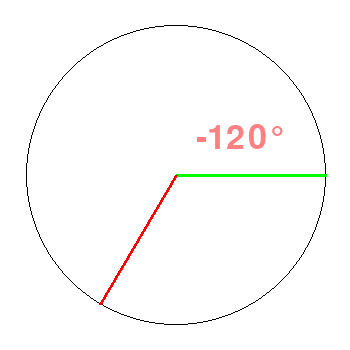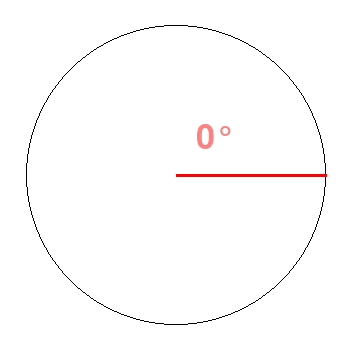
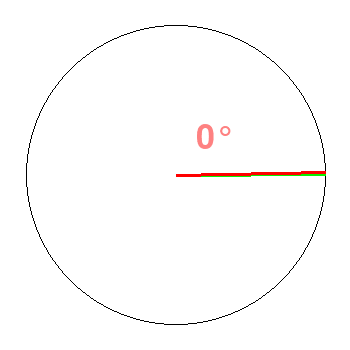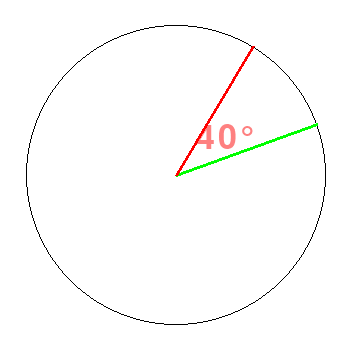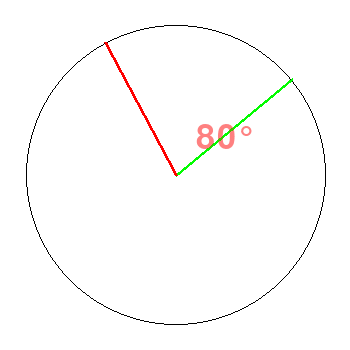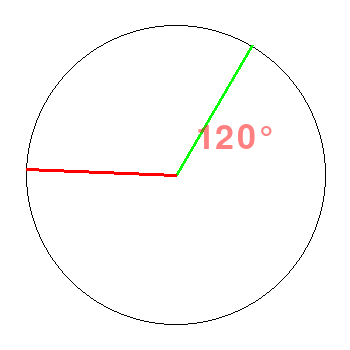
angle = acos(v1•v2)where•means "dot product"? It sounds like the two vectors here would be defined by the shooter's current location and direction the gun is currently pointing, plus the current location and the location of the enemy. – Rebarebahtangent = (y2-y1)/(x2-x1)would be used. This allows atan2 to be used. – Linkoski